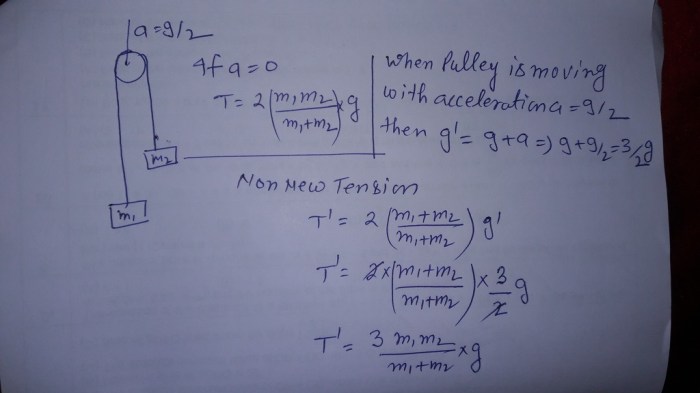
Two masses m1 and m2 are connected by a cord – Two masses, denoted as m1 and m2, connected by a cord form a captivating physical system that exhibits intriguing dynamics. This arrangement presents a rich interplay between masses, cord characteristics, and the resulting motion, offering valuable insights into fundamental principles of mechanics.
The cord connecting the masses serves as a crucial element, influencing their movement and introducing concepts such as tension and elasticity. The interplay between the masses and the cord gives rise to a complex system, where forces, energy, and motion are intricately intertwined.
Masses m1 and m2
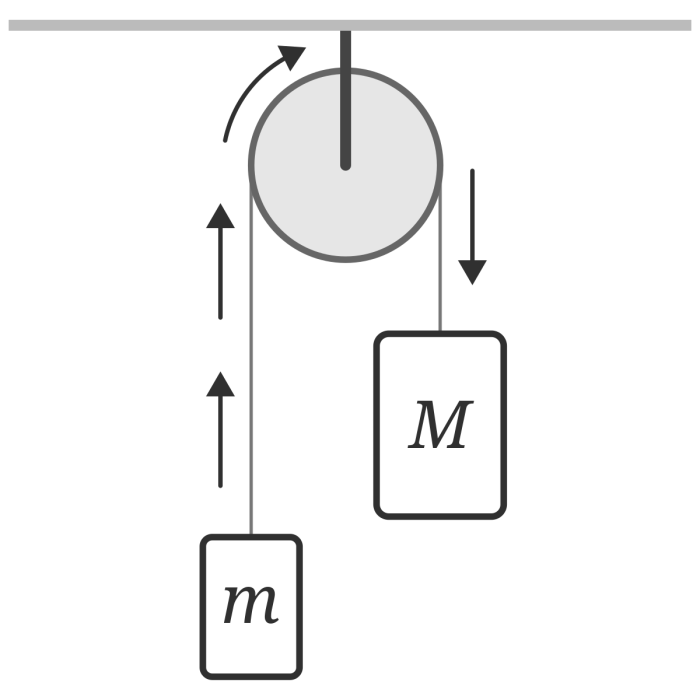
Masses m1 and m2 represent two distinct masses that are connected by a cord. The relationship between these masses is crucial in understanding the dynamics of the system they form.
The masses can be connected in various ways, such as through a pulley system, a rope, or a spring. The type of connection influences the motion and behavior of the masses.
The different masses affect the connection in several ways. A larger mass exerts a greater force on the connection, while a smaller mass experiences a smaller force. This difference in force can impact the tension and elasticity of the cord.
Cord Connection, Two masses m1 and m2 are connected by a cord
The cord connecting m1 and m2 is characterized by its length, material, and flexibility. These properties affect the motion of the masses.
The cord transmits the force between the masses, causing them to move in response to the applied force. The elasticity of the cord allows it to stretch or compress, which can influence the motion of the masses.
The tension in the cord is the force that keeps the masses connected. It arises due to the forces applied to the masses and the elasticity of the cord.
System Dynamics
The forces acting on m1 and m2 include gravity, tension in the cord, and any external forces applied to the system. These forces determine the motion of the masses.
A free body diagram can illustrate the forces acting on each mass, providing a visual representation of the system’s dynamics.
Under different conditions, the masses can move in various ways. They can accelerate, decelerate, or remain at rest, depending on the forces acting upon them.
Energy Considerations
Energy transfer occurs between m1 and m2 due to their motion. Potential energy is stored in the system when the masses are separated, and kinetic energy is generated when they move.
The concept of potential and kinetic energy is essential in understanding the energy dynamics of the system. The total energy of the system remains constant, except for energy losses due to friction or other dissipative forces.
Energy conservation analysis can provide insights into the motion and behavior of the masses.
Applications and Examples
The concepts of masses connected by a cord have numerous real-world applications. Some examples include:
- Pulleys: Lifting heavy objects by reducing the force required.
- Pendulums: Measuring time and studying periodic motion.
- Spring-mass systems: Investigating resonance and energy transfer.
These examples demonstrate the practical implications of the concepts discussed in this article.
Common Queries: Two Masses M1 And M2 Are Connected By A Cord
What factors influence the motion of masses connected by a cord?
The motion of the masses is influenced by their masses, the tension in the cord, and the external forces acting on the system.
How does the elasticity of the cord affect the system’s dynamics?
The elasticity of the cord introduces a restoring force that influences the motion of the masses, affecting their oscillation frequency and amplitude.
What is the significance of energy conservation in this system?
Energy conservation ensures that the total energy of the system remains constant, allowing us to analyze energy transfer between the masses and the cord.